| PREVIOUS PRESENTATION | BACK TO PROGRAM OVERVIEW | NEXT PRESENTATION |
THz frequency, Mie scattering of human cornea
Joel Lamberg1, Faezeh Zarrinkhat1, Roman Grigorev1,2, Irina Nefedova1,2, Juha Ala-Laurinaho1,2, Aleksi Tamminen1,2, and Zachary Taylor1,2
1Department of Electronics and Nanoengineering, Aalto University, Helsinki, Finland
2Millilab, Espoo, Finland
This talk focuses on the THz frequency scattering properties of human cornea and the effects on non-contact THz spectroscopy of corneal tissue in pursuit of corneal water content quantification. The ratio of corneal radius of curvature to illumination wavelength indicates a Mie scattering approach. Mie scattering is used to investigate where, with respect to the corneal surface, should the probing beam waist be placed. We find that focusing the beam waist on the cornea is non-optimal and that the deviation between Mie scattering results and the planar/plane wave equivalent diverge the most at low frequencies.
The cornea, the transparent front part of the eye, plays a crucial role in vision. Its optical properties are heavily influenced by its water content, which constitutes approximately 79% of its mass. Current clinical standards to assess corneal water content are limited to thickness measurements of the central cornea under the assumption that the cornea must expand to make room for increased water; water content is inferred from thickness and not measured directly. Thickness measurements do not account for the significant population variation in corneal thickness thus thickness measurements are, at best, a screening tool.
THz imaging and spectroscopy accesses the lossy longitudinal modes of the aqueous corneal shell. The tissue morphology allows for a small cavity enhancement that is leveraged for dielectric spectroscopy. The relatively simple tissue construction of cornea supports a straightforward mapping from extracted refractive index to tissue water content via effective media theory.
Typical THz spectroscopy system illuminate the cornea with a Gaussian beam oriented such that the converging beam radius of curvature is matched to the corneal radius of curvature, and parameter fit to a model that assumes plane wave interaction with a planar dielectric stack of infinite transverse extent. However, THz back scattering from cornea diverges from these assumptions and a more sophisticated scattering model is needed.
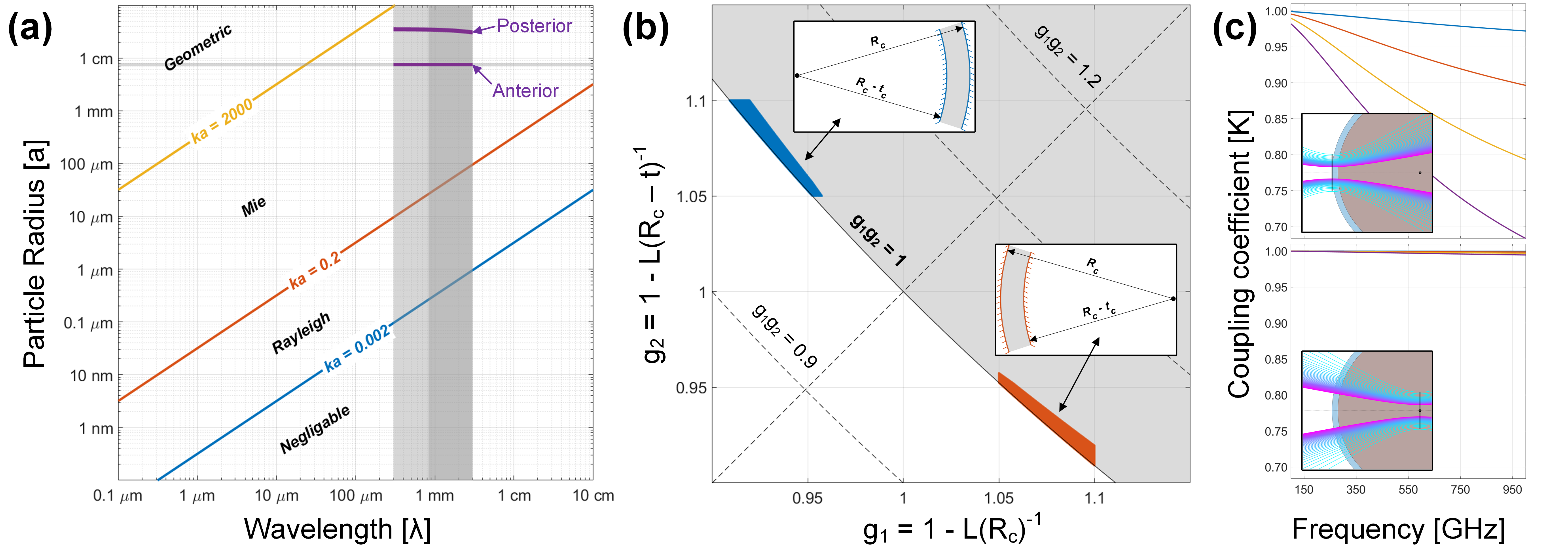
Figure 1: (a) Cornea scattering region, (b) stability analysis of the cornea as a spherical cavity. The coupling efficiency computed by ABCD matrix for beam illumination (c) on the apex, and (d) on the center of the cornea [1].
The canonical loglog scattering plot relating wavelength and particle radius is shown in Figure 1(a). Contour lines for fixed size parameter (ka = 2πn/λ) are superimposed on the plot and delineate approximate regions: “Negligible” (ka<0.002), Rayleigh (0.002 < ka < 0.2), Mie (0.2 < ka < 2000), and Geometric (ka > 2000). Regions corresponding to the anterior segment (outer cornea layer) and posterior segment (inner cornea layer) are bounded by the wavelength range corresponding to 100 – 1000 GHz, human cornea RoC of 7 – 8 mm and a central corneal thickness (CCT) range of 0.4 – 0.7 mm. Both segments lie within the Mie scattering range.
A cavity stability analysis of the cornea is shown in Figure 1(b) where beam walk-off losses are assessed by treating the cornea as a concentric spherical cavity. Concentric cavities lie directly on the g1g2 = 1. Moreover, the corneal thickness increases from center to periphery which effectively offsets the surface anterior surface, breaks the concentricity, and moves the cavity to the unstable region.
Stability/instability is further demonstrated by ABCD matrix analysis with results shown in Figure 1(c,d). A Gaussian beam was launched at the cavity and then the coupling between the initial reflection and multiple round trips were computed. For Figure 1(c), the beam waist was placed coincident with the corneal apex resulting in approximate plane wave illumination. Subsequent bounces inside the cornea reduce the radius of curvature matching as the beam diverges within the cornea. This indicates a lower sensitivity to longitudinal modes. Conversely, when the beam is placed such that the converging radius of curvature matches the corneal radius of curvature upon incidence, the coupling between initial and multipath beams is high indicating lower walk-off losses and increase sensitivity to longitudinal modes.
Exploration of these effects is performed with Fourier optics (FO), vector spherical harmonics (VSH), and the T-matrix method and the results discussed in the presentation.
References
[1] Faezeh Zarrinkhat, Joel Lamberg, Aleksi Tamminen, Mariangela Baggio, Irina Nefedova, Juha Ala-Laurinaho, Elsayed E. M. Khaled, Juan Rius, Jordi Romeu, and Zachary Taylor, “Vector spherical harmonic analysis and experimental validation of spherical shells illuminated with broadband, millimeter wave Gaussian beams: applications to corneal sensing,” Biomed. Opt. Express 13, 3699-3722 (2022)
